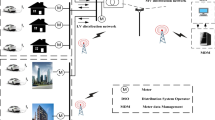
Appendix A: Linear Power Flow
In this chapter, a linear power flow is used based on [20, 30]. This power flow is used only in radial distribution networks. For this purpose, a term is considered as a block to avoid nonlinearities. Note that the EVs in the PLs act as a source at the on-peak hours and as a load at the off-peak or mid-peak hours. The active and reactive power balance in this power flow is shown in Eqs. (4.A.1) and (4.A.2). Of course in the single-level model, instead of the expected value of the charging/discharging power and the output power of the solar system in Eq. (4.A.1), their scenario values are replaced.
$$ {\displaystyle \begin{array}{l}{P}_{sb,t}^{Wh2G}\times {\eta}^{Trans}+{\hat{P}}_{PL,t}^{Solar}+\sum \limits_{EV}{\hat{P}}_{PL, EV,t}^{dch}-\sum \limits_{EV}{\hat{P}}_{PL, EV,t}^{ch}-\sum \limits_{b^{\hbox{'}}}\left[\left({P}_{b,{b}^{\hbox{'}},t,s}^{+}-{P}_{b,{b}^{\hbox{'}},t,s}^{-}\right)+{R}_{b,{b}^{\hbox{'}}}I{2}_{b,{b}^{\hbox{'}},t,s}\right]\\ {}+\sum \limits_{b^{\hbox{'}}}\left({P}_{b^{\hbox{'}},b,t,s}^{+}-{P}_{b^{\hbox{'}},b,t,s}^{-}\right)-{P}_{b,t}^{\mathrm{L}}=0\kern4.25em \forall \mathrm{t},\mathrm{s}\end{array}} $$
(4.A.1)
$$ {Q}_{sb,t}^{Wh2G}-\sum \limits_{b^{\hbox{'}}}\left[\left({Q}_{b,{b}^{\hbox{'}},t,s}^{+}-{Q}_{b,{b}^{\hbox{'}},t,s}^{-}\right)+{X}_{b,{b}^{\hbox{'}}}I{2}_{b,{b}^{\hbox{'}},t,s}\right]+\sum \limits_{b^{\hbox{'}}}\left({Q}_{b^{\hbox{'}},b,t,s}^{+}-{Q}_{b^{\hbox{'}},b,t,s}^{-}\right)-{Q}_{b,t}^{\mathrm{L},}=0\kern4em \forall \mathrm{t},\mathrm{s} $$
(4.A.2)
Note that I2 refers to an auxiliary variable linearly representing the squared current flow I2 in a given branch. At most one of these two positive auxiliary variables, i.e., Pb,b,t,s and Qb,b,t,s, can be different from zero at a time. This condition is again implicitly enforced by optimality. Moreover, Eqs. (4.A.3) and (4.A.4) limit these variables by the maximum apparent power for the sake of completeness.
$$ 0\le \left({P}_{b,{b}^{\hbox{'}},t,s}^{+}+{P}_{b,{b}^{\hbox{'}},t,s}^{-}\right)\le {V}^{Rated}\times {I}^{\max, b,b} $$
(4.A.3)
$$ 0\le \left({Q}_{b,{b}^{\hbox{'}},t,s}^{+}+{Q}_{b,{b}^{\hbox{'}},t,s}^{-}\right)\le {V}^{Rated}\times {I}^{\max, b,{b}^{\hbox{'}}} $$
(4.A.4)
Equation (4.A.5) is presented for the balancing of voltage between two nodes. It should be noted that V2 in Eq. (4.A.5) is an auxiliary variable representing the squared voltage relation.
$$ V{2}_{b,t,s}-V{2}_{b^{\hbox{'}},t,s}-{Z}_{b,{b}^{\hbox{'}}}^2I{2}_{b,{b}^{\hbox{'}},t,s}-2{R}_{b,{b}^{\hbox{'}}}\left({P}_{b,{b}^{\hbox{'}},t,s}^{+}-{P}_{b,{b}^{\hbox{'}},t,s}^{-}\right)-2{X}_{b,{b}^{\hbox{'}}}\left({Q}_{b,{b}^{\hbox{'}},t,s}^{+}-{Q}_{b,{b}^{\hbox{'}},t,s}^{-}\right)=0 $$
(4.A.5)
Equation (4.A.6) is employed for linearizing the active and reactive power flows that appear in the apparent power expression.
$$ V{2}_b^{Rated}I{2}_{b,{b}^{\hbox{'}},t,s}=\sum \limits_f\left[\left(2f-1\right)\Delta {S}_{b,{b}^{\hbox{'}}}\Delta {P}_{b,{b}^{\hbox{'}},f,t,s}\right]+\sum \limits_f\left[\left(2f-1\right)\Delta {S}_{b,{b}^{\hbox{'}}}\Delta {Q}_{b,{b}^{\hbox{'}},f,t,s}\right] $$
(4.A.6)
For the piecewise linearization, Eqs. (4.A.7), (4.A.8), (4.A.9), (4.A.10), and (4.A.11) are represented. The number of blocks required to linearize the quadratic curve is set to 10 according to [20], which strikes the right balance between accuracy and computational requirements. Further descriptions, justifications, and derivations of the network model used in this chapter can be found in [30].
$$ {P}_{b,{b}^{\hbox{'}},t,s}^{+}+{P}_{b,{b}^{\hbox{'}},t,s}^{-}=\sum \limits_f\Delta {P}_{b,{b}^{\hbox{'}},f,t,s} $$
(4.A.7)
$$ {Q}_{b,{b}^{\hbox{'}},t,s}^{+}+{Q}_{b,{b}^{\hbox{'}},t,s}^{-}=\sum \limits_f\Delta {Q}_{b,{b}^{\hbox{'}},f,t,s} $$
(4.A.8)
$$ 0\le \Delta {P}_{b,{b}^{\hbox{'}},f,t,s}\le \Delta {S}_{b,{b}^{\hbox{'}}} $$
(4.A.9)
$$ 0\le \Delta {Q}_{b,{b}^{\hbox{'}},f,t,s}\le \Delta {S}_{b,{b}^{\hbox{'}}} $$
(4.A.10)
$$ \Delta {S}_{b,{b}^{\hbox{'}}}=\frac{V^{Rated}\times {I}^{\max, b,{b}^{\hbox{'}}}}{f} $$
(4.A.11)
Appendix B: Converting the Bi-Level Model to the Single-Level Model
The presented non-linear bi-level model by using the KKT conditions and the dual theory is converted into a linear single-level model. Firstly, by using of KKT optimization conditions (which a series of equal and unequal constraints that are inherently non-linear) a single-level model will be achieved. The presence of complementary constraints is caused by the model to be non-linear. These series of constraints by the Fortuny-Amat and McCarl method which include binary variables, and a very large positive integer will be linear. Then, by using dual theory, the non-linear objective function becomes linear. When the bi-level model is converted to a single-level model, the main objective function of the final model is the linearly objective function of the upper level. Also the constraints of this model are the upper and lower level constraints, KKT’s optimization constraints and linearly KKT’s complementary constraints.
4.1.1 Converting Controlled Charging the Bi-Level Model to the Single-Level Model
At first, the constraints of the lower-level
are described as Eqs. (4.I.1), (4.I.2), (4.I.3), (4.I.4), (4.I.5), (4.I.6), (4.I.7), (4.I.8), and (4.I.9):
$$ {C}_1={SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1 $$
(4.I.1)
$$ {C}_2={P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^2 $$
(4.I.2)
$$ {C}_3={P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}_{mid/ off- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^3\kern0.5em $$
(4.I.3)
$$ {C}_4={P}_{PL, EV,{t}^{on- peak},s}^{ch- solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^4 $$
(4.I.4)
$$ {C}_5={P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{on- peak},s}^{ch- solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^5\kern0.5em $$
(4.I.5)
$$ {SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}-{SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\hbox{-} 1,\mathrm{s}}-\left(\left({P}_{PL, EV,t,s}^{ch- grid}+{P}_{PL, EV,t,s}^{ch- Solar}\right)\times {\eta}^{ch}\right)=0\kern1em \forall \mathrm{PL},\mathrm{EV},\mathrm{t}\succ {\mathrm{t}}^{arv},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\succ {\mathrm{t}}^{arv},\mathrm{s}}^6 $$
(4.I.6)
$$ {SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}-{\mathrm{SOE}}_{EV}^{\mathrm{arv}}-\left(\left({P}_{PL, EV,t,s}^{ch- grid}+{P}_{PL, EV,t,s}^{ch- Solar}\right)\times {\eta}_{ch}\right)=0\kern1em \forall \mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^7 $$
(4.I.7)
$$ {SOE}_{PL, EV,t,s}-{SOE}_{EV}^{dep}=0\kern1em \forall \mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^8 $$
(4.I.8)
$$ \sum \limits_{EV}{P}_{PL, EV,t,s}^{ch- Solar}-{P}_{PL,t,s}^{Solar}=0\kern1em \forall \mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9 $$
(4.I.9)
So, the Lagrangian function
can be achieved by Eq. (4.I.10):
$$ {\displaystyle \begin{array}{c}L=\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}\times {\Pr}_{t^{mid/ off- peak}}^{G2 PL}\\ {}-\left({SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\right)\ {\lambda}_{PL, EV,t,s}^1\\ {}-\left({P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- solar}\right)\kern0.37em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^2\\ {}-\left({P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- solar}\right)\;{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\\ {}-\left({P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{ch- solar}\right)\kern0.37em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^4-\left({P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{ch- solar}\right)\;{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5\\ {}-\left({SOE}_{PL, EV,t,s}-{SOE}_{PL, EV,t-1,s}-\left({P}_{PL, EV,t,s}^{ch- grid}\times {\eta}^{ch}\right)-\left({P}_{PL, EV,t,s}^{ch- solar}\times {\eta}^{ch}\right)\right){\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\succ {t}^{arv},\mathrm{s}}^6\ \\ {}-\left({SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{arv}}-\left({P}_{PL, EV,t,s}^{ch- grid}\times {\eta}^{ch}\right)-\left({P}_{PL, EV,t,s}^{ch- solar}\times {\eta}^{ch}\right)\right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^7\ \\ {}-\left({SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{dep}}\ \right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^8-\left(\sum \limits_{EV}{P}_{PL, EV,t,s}^{ch- solar}-{P}_{PL,t,s}^{solar}\right){\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9\end{array}} $$
(4.I.10)
Due to the decision variable in this model, KKT conditions are explained in Eqs. (4.I.11), (4.I.12), and (4.I.13):
$$ {\displaystyle \begin{array}{c}\frac{\partial L}{\partial {P}_{PL, EV,\mathrm{t},s}^{ch- grid}}={\Pr}_{t^{mid/ off- peak}}^{G2 PL}-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^2+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\\ {}+\left({\eta}_{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^6\ \left|{}_{\mathrm{t}\succ {\mathrm{t}}^{\mathrm{arv}}}\right.\right)+\left({\eta}_{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\left|{}_{t={t}^{arv}}\right.\right)=0\end{array}} $$
(4.I.11)
$$ {\displaystyle \begin{array}{c}\frac{\partial L}{\partial {P}_{PL, EV,t,s}^{ch- solar}}=-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^2+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^4+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5\\ {}+\left({\eta}^{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^6\ \left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.\right)+\left({\eta}^{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\left|{}_{t={t}^{arv}}\right.\right)-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9=0\end{array}} $$
(4.I.12)
$$ {\displaystyle \begin{array}{c}\frac{\partial L}{SOC_{PL, EV,t,s}}={\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1+{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}+1,\mathrm{s}}^6\left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^6\left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.\\ {}-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\left|{}_{\mathrm{t}={\mathrm{t}}_{\mathrm{arv}}}\right.-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^8\left|{}_{\mathrm{t}={\mathrm{t}}_{\mathrm{dep}}}\right.=0\end{array}} $$
(4.I.13)
The dual variables of unequal constraints are equal or greater than zero, and the dual variables whose constraints are equal to zero are unrestricted in sign. For Eqs. (4.I.1), (4.I.2), (4.I.3), (4.I.4) and (4.I.5), the complementary constraints are as follows, i.e. Eqs. (4.I.14), (4.I.15), (4.I.16), (4.I.17), and (4.I.18).
$$ 0\le {SOE}_{EV}^{\mathrm{max}}\hbox{-} {SOE}_{PL, EV,t,s}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1\kern0.5em \ge 0 $$
(4.I.14)
$$ 0\le {P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^2\ge 0 $$
(4.I.15)
$$ 0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\ge 0 $$
(4.I.16)
$$ 0\le {P}_{PL, EV,{t}^{on- peak},s}^{ch- solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^4\ge 0 $$
(4.I.17)
$$ 0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{on- peak},s}^{ch- solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^5\ge 0 $$
(4.I.18)
The linearization of complementary constraints is performed by Fortuny-Amat and McCarl linearization method by Eq. (4.I.19) [21]. Then, Eqs. (4.I.20), (4.I.21), (4.I.22), (4.I.23), and (4.I.24) are obtained.
$$ {\displaystyle \begin{array}{l}0\le {F}_1\perp {F}_2\ge 0\\ {}0\le {F}_1\le U\times M\\ {}0\le {F}_2\le \left(1-U\right)\times M\\ {} U\varepsilon \left[0,1\right]\kern0.24em \end{array}} $$
(4.I.19)
$$ {\displaystyle \begin{array}{l}0\le {SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\le {U}_{PL, EV,t,s}^1\times {M}^1\\ {}0\le {\lambda}_{PL, EV,t,s}^1\le \left(1-{U}_{PL, EV,t,s}^1\right)\times {M}^2\;\end{array}} $$
(4.I.20)
$$ {\displaystyle \begin{array}{l}0\le {P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- solar}\le {U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^2\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^2\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^2\right)\times {M}^2\kern0.24em \end{array}} $$
(4.I.21)
$$ {\displaystyle \begin{array}{l}0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- solar}\le {U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^3\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^3\right)\times {M}^2\end{array}} $$
(4.I.22)
$$ {\displaystyle \begin{array}{l}0\le {P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{ch- solar}\le {U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^4\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^4\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^4\right)\times {M}^2\kern0.24em \end{array}} $$
(4.I.23)
$$ {\displaystyle \begin{array}{l}0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{ch- solar}\le {U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^5\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^5\right)\times {M}^2\end{array}} $$
(4.I.24)
The obtained model is a non-linear single-level model, which must be linearized using the dual theory. So, firstly, the dual objective function of the lower-level model is formed as Eq. (4.I.25):
$$ {\displaystyle \begin{array}{l}\operatorname{Maximize}\\ {}+\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left(\begin{array}{l}-\left({SOE}_{EV}^{\mathrm{max}}\times {\lambda}_{PL, EV,t,s}^1\right)-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}_{mid/ off- peak},\mathrm{s}}^3\right)\\ {}-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^5\right)+\left({\mathrm{SOE}}_{EV}^{\mathrm{arv}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^7\right)\\ {}+\left({\mathrm{SOE}}_{EV}^{\mathrm{dep}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^8\right)+\left({P}_{PL,t,s}^{Solar}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9\right)\end{array}\right)\end{array}} $$
(4.I.25)
According to the strong dual theory, the objective functions of the original and dual problems are equal at the optimal point of the decision variables of the two problems; therefore, the non-linear section of the objective function is linear according to Eq. (4.I.26).
$$ {\displaystyle \begin{array}{l}\;\sum \limits_{s=1}^{Ns}{\rho}_s\left(\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{n=1}^N\sum \limits_{t=1}^{24}{P}_{PL,n,{\mathrm{t}}^{mid/ off- peak},s}^{ch}\times {\Pr}_{{\mathrm{t}}^{mid/ off- peak}}^{G2 PL}\ \right)=\\ {}\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left({\hat{P}}_{PL, EV,{t}^{mid/ off- peak}}^{ch- grid}\times {\Pr}_{t^{mid/ off- peak}}^{G2 PL}\right)=\\ {}=\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left(\begin{array}{l}-\left({SOE}_{EV}^{\mathrm{max}}\times {\lambda}_{PL, EV,t,s}^1\right)-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\right)\\ {}-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^5\right)+\left({\mathrm{SOE}}_{EV}^{\mathrm{arv}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^7\right)\\ {}+\left({\mathrm{SOE}}_{EV}^{\mathrm{dep}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^8\right)+\left({P}_{PL,t,s}^{Solar}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9\right)\end{array}\right)\end{array}} $$
(4.I.26)
4.1.2 Converting Charging/Discharging Schedule the Bi-Level Model to the Single-Level Model
At first, the constraints of the lower-level are described as Eqs. (4.II.1), (4.II.2), (4.II.3), (4.II.4), (4.II.5), (4.II.6), (4.II.7), (4.II.8), (4.II.9), and (4.II.10):
$$ {C}_1={SOE}_{PL, EV,t,s}-{SOE}_{EV}^{\mathrm{min}}\ge 0\kern1.25em \forall \mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1 $$
(4.II.1)
$$ {C}_2={SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^2\kern0.5em $$
(4.II.2)
$$ {C}_3={P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^3 $$
(4.II.3)
$$ {C}_4={P}^{\mathrm{max}}-{P}_{PL, EV,{t}_{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^4\kern0.5em $$
(4.II.4)
$$ {C}_5={P}_{PL, EV,{t}^{on- peak},s}^{dch}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^5 $$
(4.II.5)
$$ {C}_6={P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{on- peak},s}^{dch}\ge 0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{on- peak},\mathrm{s}}^6 $$
(4.II.6)
$$ {SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}-{SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\hbox{-} 1,\mathrm{s}}+\left(\frac{P_{PL, EV,t,s}^{dch}}{\eta^{dch}}\right)-\left(\left({P}_{PL, EV,t,s}^{ch- grid}+{P}_{PL, EV,t,s}^{ch- Solar}\right)\times {\eta}^{dch}\right)=0\kern1em \forall \mathrm{PL},\mathrm{EV},\mathrm{t}\succ {\mathrm{t}}^{arv},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\succ {\mathrm{t}}^{arv},\mathrm{s}}^7 $$
(4.II.7)
$$ {SOE}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}-{\mathrm{SOE}}_{EV}^{\mathrm{arv}}+\left(\frac{P_{PL, EV,t,s}^{dch}}{\eta^{dch}}\right)-\left(\left({P}_{PL, EV,t,s}^{ch- grid}+{P}_{PL, EV,t,s}^{ch- Solar}\right)\times {\eta}^{ch}\right)=0\kern1em \forall \mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^8 $$
(4.II.8)
$$ {SOE}_{PL, EV,t,s}-{SOE}_{EV}^{dep}=0\kern1em \forall \mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}\kern1em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^9 $$
(4.II.9)
$$ \sum \limits_{EV}{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\kern1em -{P}_{PL,{t}^{mid/ off- peak},s}^{Solar}=0\kern1em \forall \mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}\kern1em {\uplambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^{10} $$
(4.II.10)
Based on the previous part, Eqs. (4.II.11), (4.II.12), (4.II.13), (4.II.14), (4.II.15), (4.II.16), (4.II.17), (4.II.18), (4.II.19), (4.II.20), (4.II.21), (4.II.22), (4.II.23), (4.II.24), (4.II.25), (4.II.26), and (4.II.27) is showing the single-level steps:
$$ {\displaystyle \begin{array}{l}L=\\ {}\sum \limits_{s=1}^{Ns}{\rho}_s\left(\begin{array}{l}\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- grid}\times {\Pr}_{{\mathrm{t}}^{mid/ off- peak}}^{G2 PL}\ \\ {}+\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}{P}_{PL, EV,{t}^{on- peak},s}^{dch}\times \left(0.5{\Pr}_t^{PL2G}+{C}^{cd}\right)\end{array}\right)\\ {}-\left({SOE}_{PL, EV,t,s}-{SOE}_{EV}^{\mathrm{min}}\right)\ {\lambda}_{PL, EV,t,s}^1\\ {}-\left({SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\right)\ {\lambda}_{PL, EV,t,s}^2\\ {}-\left({P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\right)\kern0.37em {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\\ {}-\left({P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}\right)\;{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\\ {}-\left({P}_{PL, EV,{t}^{on- peak},s}^{dch}\right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5-\left({P}^{\mathrm{max}}-{P}_{PL, EV,{t}^{on- peak},s}^{dch}\right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\\ {}-\left(\begin{array}{l}{SOE}_{PL, EV,t,s}-{SOE}_{PL, EV,t-1,s}-\left({P}_{PL, EV,t,s}^{ch- grid}\times {\eta}^{ch}\right)\\ {}-\left({P}_{PL, EV,t,s}^{ch- Solar}\times {\eta}^{ch}\right)+\left(\frac{P_{PL, EV,t,s}^{dch}}{\eta_{dch}}\right)\ \end{array}\right){\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}\succ {t}^{arv},\mathrm{s}}^7\ \\ {}-\left(\begin{array}{l}{SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{arv}}-\left({P}_{PL, EV,t,s}^{ch- grid}\times {\eta}^{ch}\right)\\ {}-\left({P}_{PL, EV,t,s}^{ch- Solar}\times {\eta}^{ch}\right)+\left(\frac{P_{PL, EV,t,s}^{dch}}{\eta^{dch}}\right)\end{array}\right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^8\ \\ {}-\left({SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{dep}}\ \right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^9\\ {}-\left(\sum \limits_{EV}{P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- Solar}-{P}_{PL,{t}^{mid/ off- peak},s}^{Solar}\right){\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^{10}\end{array}} $$
(4.II.11)
$$ {\displaystyle \begin{array}{l}\frac{\partial L}{\partial {P}_{PL, EV,t,s}^{ch- grid}}={\Pr}_{{\mathrm{t}}^{mid/ off- peak}}^{G2 PL}-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\\ {}+\left({\eta}^{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\ \left|{}_{\mathrm{t}\succ {\mathrm{t}}^{\mathrm{arv}}}\right.\right)+\left({\eta}^{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^8\left|{}_{t={t}^{arv}}\right.\right)=0\end{array}} $$
(4.II.12)
$$ {\displaystyle \begin{array}{l}\frac{\partial L}{\partial {P}_{PL, EV,t,s}^{ch- Solar}}=-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4+\left({\eta}_{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\ \left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.\right)\\ {}+\left({\eta}^{ch}\times {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^8\left|{}_{t={t}^{arv}}\right.\right)-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^{10}=0\end{array}} $$
(4.II.13)
$$ {\displaystyle \begin{array}{l}\frac{\partial L}{P_{PL, EV,t,s}^{dch}}=0.5{\Pr}_{{\mathrm{t}}_{on- peak}}^{PL2G}+{C}^{cd}-\left(\frac{\lambda_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7}{\eta^{dch}}\ \left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.\right)-\left(\frac{\lambda_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^8}{\eta^{dch}}\ \left|{}_{\mathrm{t}={\mathrm{t}}_{\mathrm{arv}}}\right.\right)\\ {}-{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}_{on- peak},\mathrm{s}}^5+{\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6=0\end{array}} $$
(4.II.14)
$$ {\displaystyle \begin{array}{l}\frac{\partial L}{SOC_{PL, EV,t,s}}={\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t}+1,\mathrm{s}}^7\left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^7\left|{}_{\mathrm{t}\succ {\mathrm{t}}_{\mathrm{arv}}}\right.-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^8\left|{}_{\mathrm{t}={\mathrm{t}}_{\mathrm{arv}}}\right.\\ {}-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^9\left|{}_{\mathrm{t}={\mathrm{t}}_{\mathrm{dep}}}\right.-{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1+{\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^2=0\end{array}} $$
(4.II.15)
$$ 0\le {SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{min}}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^1\ge 0\kern0.72em $$
(4.II.16)
$$ 0\le {\mathrm{SOE}}_{EV}^{\mathrm{max}}-{SOC}_{PL, EV,t,s}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},\mathrm{t},\mathrm{s}}^2\kern0.5em \ge 0 $$
(4.II.17)
$$ 0\le {P}_{PL, EV,{\mathrm{t}}_{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- Solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}_{mid/ off- peak},\mathrm{s}}^3\ge 0 $$
(4.II.18)
$$ 0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- Solar}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\ge 0 $$
(4.II.19)
$$ 0\le {P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{dch}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5\ge 0 $$
(4.II.20)
$$ 0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{dch}\perp {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\kern0.36em \ge 0 $$
(4.II.21)
$$ {\displaystyle \begin{array}{l}0\le {SOE}_{PL, EV,t,s}-{\mathrm{SOE}}_{EV}^{\mathrm{min}}\le {U}_{PL, EV,t,s}^1\times {M}^1\\ {}0\le {\lambda}_{PL, EV,t,s}^1\le \left(1-{U}_{PL,n,t,s}^1\right)\times {M}^2\;\end{array}} $$
(4.II.22)
$$ {\displaystyle \begin{array}{l}0\le {SOE}_{EV}^{\mathrm{max}}-{SOE}_{PL, EV,t,s}\le {U}_{PL,n,t,s}^2\times {M}^1\\ {}0\le {\lambda}_{PL, EV,t,s}^2\le \left(1-{U}_{PL,n,t,s}^2\right)\times {M}^2\;\end{array}} $$
(4.II.23)
$$ {\displaystyle \begin{array}{l}0\le {P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- grid}+{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- Solar}\le {U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^3\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^3\le \left(1-{U}_{PL,n,{\mathrm{t}}^{mid/ off- peak},s}^3\right)\times {M}^2\kern0.24em \end{array}} $$
(4.II.24)
$$ {\displaystyle \begin{array}{l}0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}_{mid/ off- peak},s}^{ch- grid}-{P}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^{ch- Solar}\le {U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^4\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{mid/ off- peak},s}^4\right)\times {M}^2\end{array}} $$
(4.II.25)
$$ {\displaystyle \begin{array}{l}0\le {P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{dch}\le {U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^5\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^5\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^5\right)\times {M}^2\kern0.24em \end{array}} $$
(4.II.26)
$$ {\displaystyle \begin{array}{l}0\le {P}^{\mathrm{max}}-{P}_{PL, EV,{\mathrm{t}}^{on- peak},s}^{dch}\le {U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^6\times {M}^1\\ {}0\le {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\le \left(1-{U}_{PL, EV,{\mathrm{t}}^{on- peak},s}^6\right)\times {M}^2\end{array}} $$
(4.II.27)
The non-linear part of the objective function can be converted to a linear part with two equations i.e. (4.II.28) and (4.II.29).
$$ {\displaystyle \begin{array}{l}\mathit{\operatorname{Maximize}}\\ {}\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left(\begin{array}{l}\left({SOE}_{EV}^{\mathrm{min}}\times {\lambda}_{PL, EV,t,s}^1\right)-\left({SOE}_{EV}^{\mathrm{max}}\times {\lambda}_{PL, EV,t,s}^2\right)\\ {}-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\right)-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\right)\\ {}+\left({\mathrm{SOE}}_{EV}^{\mathrm{arv}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^8\right)+\left({\mathrm{SOE}}_{EV}^{\mathrm{dep}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^9\right)\\ {}+\left({P}_{PL,{t}^{mid/ off- peak},s}^{Solar}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^{10}\right)\end{array}\right)\end{array}} $$
(4.II.28)
$$ {\displaystyle \begin{array}{l}\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{n=1}^N\sum \limits_{t=1}^{24}\left(\begin{array}{l}\left({P}_{PL,n,{t}^{mid/ off- peak},s}^{ch- grid}\times {\Pr}_{t^{mid/ off- peak}}^{G2 PL}\right)\\ {}+\left({P}_{PL,n,{t}^{on- peak},s}^{dch}\times \left(0.5{\Pr}_{t^{on- peak}}^{PL2G}+{C}^{cd}\right)\right)\end{array}\right)\\ {}=\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left(\begin{array}{l}\left({SOE}_{EV}^{\mathrm{min}}\times {\lambda}_{PL, EV,t,s}^1\right)-\left({SOE}_{EV}^{\mathrm{max}}\times {\lambda}_{PL, EV,t,s}^2\right)\\ {}-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\right)-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\right)\\ {}+\left({\mathrm{SOE}}_{EV}^{\mathrm{arv}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^8\right)+\left({\mathrm{SOE}}_{EV}^{\mathrm{dep}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^9\right)\\ {}+\left({P}_{PL,{t}^{mid/ off- peak},s}^{Solar}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^{10}\right)\end{array}\right)\\ {}\\ {} So:\\ {}\sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}{P}_{PL, EV,{t}^{on- peak},s}^{dch}\times {\Pr}_{t^{on- peak}}^{PL2G}=\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left({\hat{P}}_{PL, EV,{t}^{mid/ off- peak}}^{dch}\times {\Pr}_{t^{mid/ off- peak}}^{G2 PL}\right)=\\ {}=2\times \sum \limits_{s=1}^{Ns}{\rho}_s\sum \limits_{PL=1}^{N_{PL}}\sum \limits_{EV=1}^{N_{EV}}\sum \limits_{t=1}^{24}\left(\begin{array}{l}\left({SOE}_{EV}^{\mathrm{min}}\times {\lambda}_{PL, EV,t,s}^1\right)-\left({SOE}_{EV}^{\mathrm{max}}\times {\lambda}_{PL, EV,t,s}^2\right)\\ {}-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{mid/ off- peak},\mathrm{s}}^4\right)-\left({P}^{\mathrm{max}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{on- peak},\mathrm{s}}^6\right)\\ {}+\left({\mathrm{SOE}}_{EV}^{\mathrm{arv}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{arv},\mathrm{s}}^8\right)+\left({\mathrm{SOE}}_{EV}^{\mathrm{dep}}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{\mathrm{t}}^{dep},\mathrm{s}}^9\right)\\ {}+\left({P}_{PL,{t}^{mid/ off- peak},s}^{Solar}\times {\lambda}_{\mathrm{PL},\mathrm{EV},{t}^{mid/ off- peak},\mathrm{s}}^{10}\right)-\left({P}_{PL, EV,{t}^{on- peak},s}^{dch}\times {C}^{cd}\right)\\ {}-\left({P}_{PL, EV,{t}^{mid/ off- peak},s}^{ch- grid}\times {\Pr}_{t^{mid/ off- peak}}^{G2 PL}\right)\end{array}\right)\end{array}} $$
(4.II.29)
Appendix C
The nomenclature is shown below.
Indices | |
|---|
b, b′ | Index for branch or bus |
EV | Index for EV number |
F | Index for linear partitions in linearization |
s | Index for scenarios |
sb | Index for slack bus |
t, t′ | Index for time (hour) |
Parameters |
Ccd | Cost of equipment depreciation ($/kWh) |
Imax | Upper limit of branches’ current (A) |
Imax, b, b′ | Maximum current of branch b, b′ (A) |
M | Sufficiently large constants |
PL | The demand of customers (kW) |
Pmax | Nominal rate of charging/discharging of EVs (kWh) |
PSolar | Power generated of the solar system (kW) |
PrL | Electricity price for the customer ($/kWh) |
PrWh2G | The wholesale market electricity price ($/kWh) |
R b, b′ | Resistance between branch b, b′ (Ω) |
QL | Customer’s reactive power (kVAR) |
SOEarv | Initial SOE of the EVs (kWh) |
SOEdep | Desired SOE of the EVs (kWh) |
SOEmax | Upper limit of SOE (kWh) |
SOEmin | Lower limit of SOE (kWh) |
tarv | Arrival time of the EVs to the PL |
tdep | Departure time of the EVs from the PL |
V Rated | Nominal voltage (V) |
Vmax | Maximum allowable voltage (V) |
Vmin | Minimum allowable voltage (V) |
X b, b′ | Reactance between branch b, b′ (Ω) |
Z | Impedance (Ω) |
ηch | Charging efficiency (%) |
ηdch | Discharging efficiency (%) |
ηTrans | Transformer efficiency (%) |
ρ | Probability of each scenario |
α | Confidence level |
β | Risk aversion parameter |
ΔS | Upper limit in the discretization of quadratic flow terms (kVA) |
Variables | |
B | Profit in each scenario |
I,I2 | Current flow (A), squared current flow (A2) |
Pch-grid | Charging power of the EVs by the SDNO (kW) |
Pch-solar | Charging power of the EVs by the power generated of the solar system (kW) |
\( {\hat{P}}^{ch- grid} \) | The expected value of charging power of the EVs by the SDNO (kW) |
\( {\hat{P}}^{solar} \) | The expected value of the power generated of the solar system (kW) |
Pdch | Discharging power of the EVs (kW) |
\( {\hat{P}}^{dch} \) | The expected value of discharging power of the EVs (kW) |
PLoss | SDN’s losses (kW) |
PWh2G | Purchasing power from the wholesale by the SDNO (kW) |
P+ | Active power flows in downstream directions (kW) |
P− | Active power flows in upstream directions (kW) |
PrG2PL | Charging tariff of the EVs ($/kWh) |
PrPL2G | Discharging tariff of the EVs ($/kWh) |
QWh2G | SDN’s reactive power (kVAR) |
Q+ | Reactive power flows in downstream directions (kVAR) |
Q− | Reactive power flows in upstream directions (kVAR) |
SOE | State of energy (kWh) |
U | Binary variable |
λ | Dual variable ($/kWh) |
η | Auxiliary variable for calculating CVaR |
ξ | Value-at-risk |